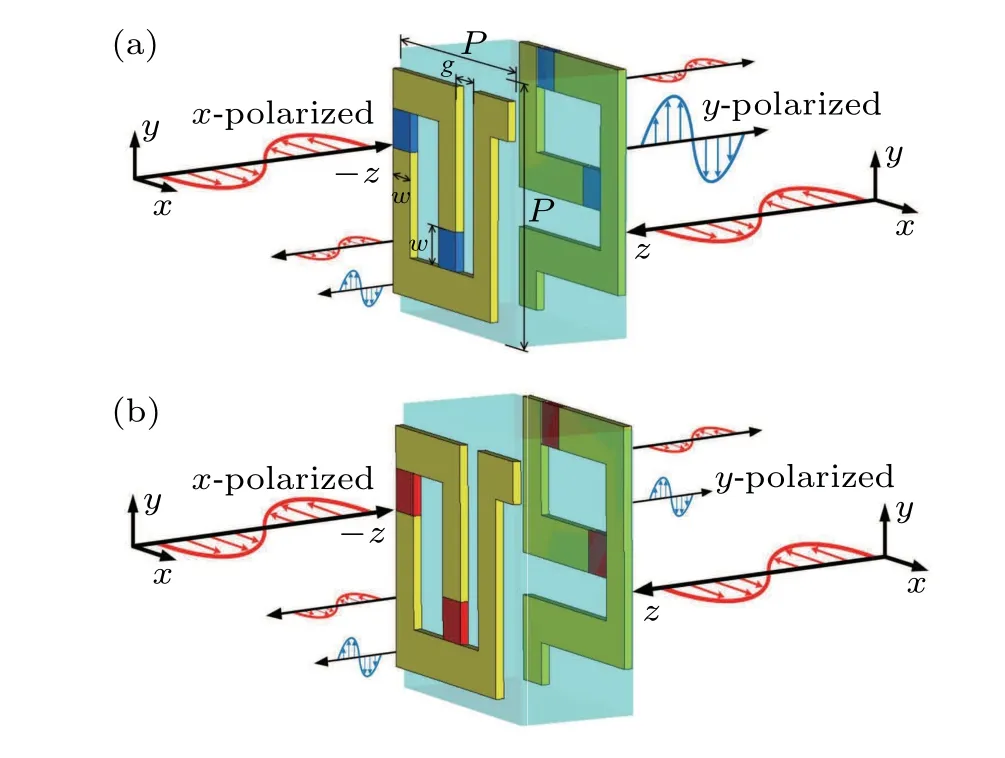
Dynamically controlled asymmetric transmission of linearly polarized waves in VO2-integrated Dirac semimetal metamaterials
Man Xu(許曼) Xiaona Yin(殷曉娜) Jingjing Huang(黃晶晶) Meng Liu(劉蒙)Huiyun Zhang(張會云) and Yuping Zhang(張玉萍)
1College of Electronic and Information Engineering,Shandong University of Science and Technology,Qingdao 266590,China
2Collaborative Innovation Center of Light Manipulations and Applications,Shandong Normal University,Jinan 250358,China
Keywords: terahertz,chiral metamaterials,asymmetric transmission,tunable
1. Introduction
Terahertz (THz) waves lie between microwave and infrared waves in the electromagnetic spectrum, and they have many unique properties,[1]including high signal-noise ratio,ultra-wideband, and strong transmission capability.[2]Natural materials have a relatively weak electromagnetic response in the terahertz band. Therefore, traditional terahertz modulators are not only inefficient, but also very thick, which is not conducive to the development of modern optical systems.Metamaterials manipulate terahertz wave at will by the arbitrary design of subwavelength microstructure array. They have been realized many novel electromagnetic effects which are not available from conventional materials,such as negative magnetic permeability,[3,4]zero refractive index,[5,6]and negative refractive index.[7]The introduction of terahertz metamaterial has promoted the development of high efficiency and integrated modulators, which have filled the “THz gap” to a large extent.[8]Asymmetric transmission(AT)devices are one of the branches of terahertz functional devices,which exhibit promising application in the development of radomes,circulators and isolators.
In 2006,Fedotovet al.[9]proposed AT of circularly polarized waves for the first time by using fishscale-shaped metamaterials in the optical frequency. Then in 2010, Menzelet al.[10]first realized AT of linearly polarized waves in threedimensional(3D)chiral optical metamaterials. Subsequently,chiral metamaterials have been widely used to achieve AT of linearly polarized waves in the microwave[11]and terahertz regions.[12]In 2017, Fanget al.[13]proposed a dualband AT device composed of five-layer metamaterial structure,which achieved strong AT effect for the linearly polarized incidence around 0.51 THz and 0.81 THz. In 2018, Stephenet al.[14]proposed a sandwich structure for AT of linearly polarized waves with a maximum AT parameter of 0.95 in the microwave band. However,once these devices have been fabricated,their working frequency and amplitude cannot be controlled in real time, which seriously limits their practical application.
In order to overcome the above bottlenecks and obtain THz devices with dynamically controlled performance, materials with adjustable electromagnetic properties are combined into metamaterial structures, such as graphene,[15–19]Dirac semimetals(DSMs),[20]and vanadium dioxide (VO2).[21–24]These tunable metamaterial structures are conducive to the development of novel terahertz devices,such as modulators,[25–28]filters,[25–28]and sensors.[25–27]Zhaoet al.[15]proposed a three-layer metal–graphene–metal metasurface to achieve the tunability of linear AT by varying the Fermi energy of the graphene.However,graphene exhibits an instable property and interact light weakly since it is composed of single layer atom,[29]which limits its development of high efficiency AT devices. DSMs are considered to be analogues of three-dimensional graphene. In comparison, it has higher electron mobility[30,31]and interacts with incident waves strongly.[32]Furthermore, the Fermi energy of DSMs can be by alkaline surface doping.[33,34]These excellent properties make DSMs a suitable candidate material for the construction of actively controlled metamaterial absorbers,[35–38]electromagnetic induced transparency[39–43]devices,polarization conversion devices.[44,45]Daiet al.[20]designed AT devices for linearly polarized waves based on DSMs. The proposed device achieves a maximum AT parameter of 0.38 at 1.56 THz,and the resonant frequency and amplitude were dynamically tunable by changing the DSMs Fermi energy. However,the tunability of the resonant frequency was accompanied by a reduction in the AT intensity as the decrease of the DSMs Fermi level. It is well-known that VO2exhibits a phase transition from an insulating dielectric state to a metallic state[42]via electrical,thermal,and optical stimuli.[46–48]During the transition progress, VO2undergoes a drastic change in conductivity exceeding four orders of magnitude around the critical temperature 68°. Recently,Liuet al.[21]carried out a systematic research on temperature-controlled two-dimensional(2D)chirality and 3D chirality in the terahertz regime,including the dynamically controlled linearly polarized AT,[22]switchable chiral mirrors,[23]and actively controlled negative refractive index.[24]However, all AT of linearly polarized devices were designed cannot simultaneously realize these two functionalities. Firstly, the resonant frequency of the AT is dynamically tunable while maintaining the amplitude of AT.Then,the amplitude can be dynamically tuned independently leading to a switch for AT.
In this work, we proposed a theoretical AT device based on VO2and DSMs in the terahertz region. At the room temperature,VO2is in its insulating state,tunable broadband AT of linearly polarized terahertz waves has been achieved. The resonant frequency can be dynamically tuned by modifying the Fermi level of DSFs while maintaining the amplitude of AT. Increasing the operation temperature across 68°C, VO2transmit from insulating state to metallic state, the AT amplitude of linearly polarized waves decrease significantly, while the resonant frequency remains the same almost be almost unchanged. This work can be used to inspire the fabrication of tunable AT devices in the terahertz range,as well as the similar modulator operating in other frequency.
2. Design and theoretical analysis
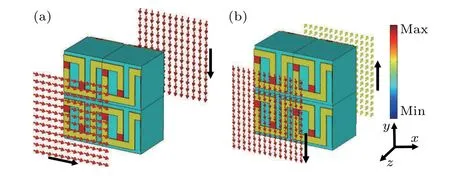
Figure 1 illustrates the schematic of a unit cell of the proposed chiral metasurface,which consists of three layers. The top layer composed of DSMs and VO2microstructures,which a 6-shaped structure with a thickness of 0.7 μm. The 6-shaped patterns on the front and back surfaces of the dielectric layer are structurely identical and the bottom pattern is a mirror image of the top layer rotated by 90°. The polyimide layers have been treated as a lossy material with dielectric constant set toεp=3+0.09i,[49]and its thickness is 21 μm. The width of DSMs bars isw=3 μm, and the gap between DSMs bars isg=3 μm. The period of the unit isP=20 μm. When VO2in its insulating state, this chiral metasurface can achieve AT effect shown in Fig. 1(a). VO2transmit from insulating state to metallic state,AT can be turned off shown in Fig.1(b). The electromagnetic simulation software CST Microwave Studio is used to simulate the chiral metasurface. In simulations,the unit cell boundary conditions are employed along thexandydirections, and the open boundary condition is applied along thezdirection.
In the range of long wave limitq ?KF, the longitudinal dynamic conductivity formula of DSMs can be obtained by Kubo formula in random-phase approximation theory. The conductivity of DSMs can be calculated by the formulaδ(w)=δintra(w)+δinter(w),whereδintra(w)andδinter(w)are the intraband and interband conductivity respectively. The interband and intraband conductivity of the DSMs can be defined as[50]
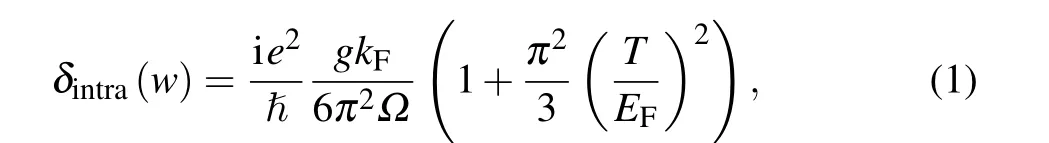
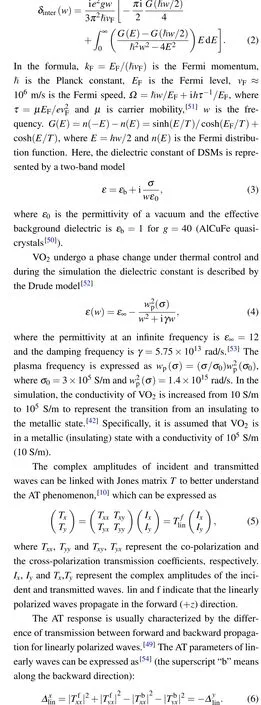
Since the 6-shaped patterns on the front and back surfaces of the dielectric layer have the characteristic of chirality,the copolarized transmission coefficients of linearly polarized waves are always equal. The formula can be further simplified as
3. Results and discussion
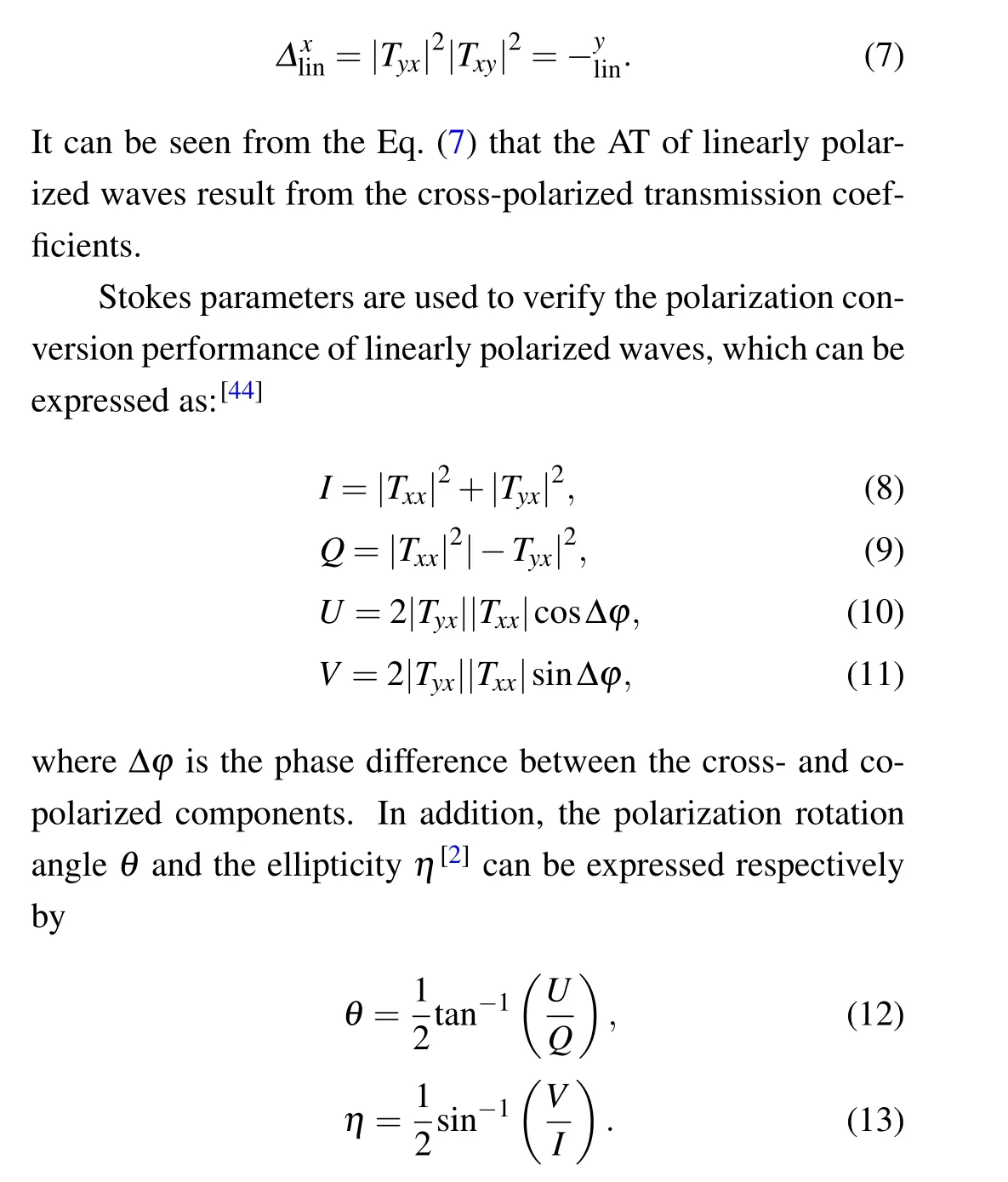
The spectra response of the proposed device has been demonstrated for the case where VO2is in the insulating dielectric state with conductivity of 10 S/m, and the Fermi energy level of the DSMs is 0.15 eV.Figures 2(a)and 2(b)show the four elements of the transmission matrix for a linearly polarized wave propagating along the backward (-z) and forward(+z)directions.For they-polarized waves propagating in the backward direction and thex-polarized waves propagating in the forward direction,the AT device allows waves to transmit through themselves with a perfect diode effect. As shown in Fig.2(a),the co-polarization transmission coefficients(Txx,Tyy)are always equal over the entire frequency range,while the cross-polarization transmission coefficients (Txy,Tyx) are significantly different. The value of|Tyx| is higher than 0.7 and|Txy|is lower than 0.16 in the range of 1.184 THz–1.8336 THz.Compared with Figs.2(a)and 2(b),when the propagation direction is switched,the co-polarized transmission coefficients remains unchanged, while cross-polarized transmission coefficients will exchange. It can be observed from Figs.2(c)and 2(d), when thex-linearly polarized waves propagating along the backward direction, the polarization azimuth is 88.1°and-68.8°at 1.184 THz and 1.8336 THz. Therefore, these phenomena are identical to the conditions required for the AT effect that cause AT of linearly polarized waves.

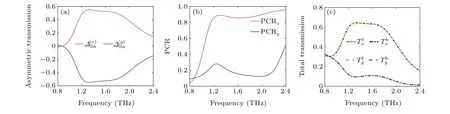
The AT performance can be described more intuitively by introducing the polarization conversion ratio (PCR) and the total transmission intensity (T) of thex- andy-linearly polarized waves. The equations PCRx=|Tyx|2/(|Txx|2+|Tyx|2)and PCRy=|Txy|2/(|Tyy|2+|Txy|2) are used to illustrate the performance of thex- andy-linear PCRxand PCRyshown in Fig.3(b). PCRxis greater than 0.8 in the range of 1.15 THz–2.4 THz, indicating that most of thex-polarized waves are converted toy-polarized waves. The equations|Tx|2=|Txx|2+|Tyx|2and|Ty|2=|Tyy|2+|Txy|2represent the total transmittance ofx-andy-polarized waves. Figure 3(c)shows the total transmission intensity of linearly polarized waves propagating from the forward and backward directions respectively. Total transmission intensityTbxofx-polarized waves incident along backward direction shows one peak of 0.64 at 1.33 THz,while total transmission intensityTbyofy-polarized waves is relatively low.
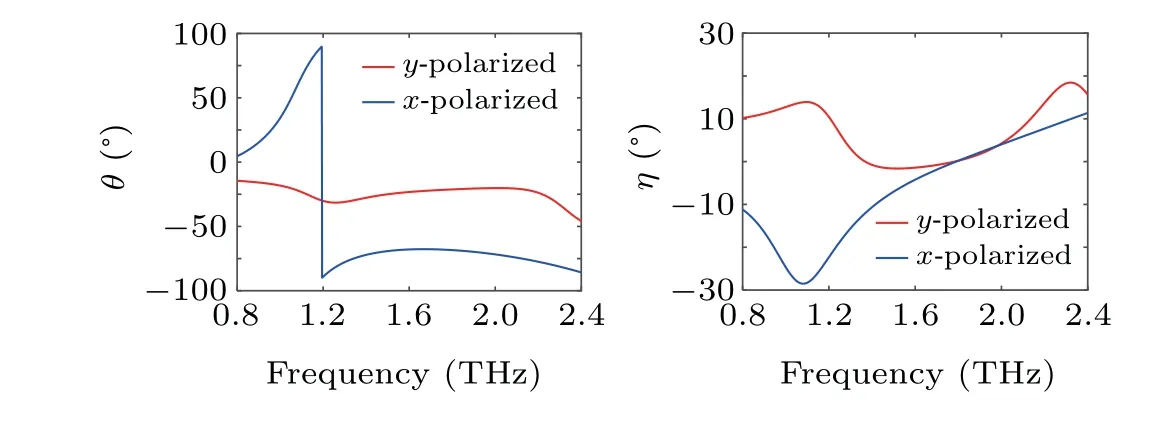
According to Eqs. (12) and (13), we calculate the polarization rotation angleθand ellipticityηof linearly polarized waves propagating in the backward direction shown in Figs.4(a)and 4(b). As can be seen from Fig.4(a),the polarization azimuth of thex-polarized waves at 1.2 THz is close to 90°,achieving a perfectx–ypolarization conversion. However,the absolute value ofy-polarized waves in the whole frequency range is less than 25°,so it can hardly be converted intox-polarized waves when passing through the device. The incident linearly polarized waves change into linearly or elliptically polarized waves after passing through the chiral structure as shown in Fig.4(b). Theηofx-polarized andy-polarized is 0 at the frequency 1.79 THz,which indicate that the transmitted wave is still linearly polarized. In the frequency range of less than 1.79 THz, theηofx-polarized wave is less than 0,thus the transmitted wave becomes right-handed elliptical polarized one. When the frequency is greater than 1.79 THz,ηis always more than 0,and the transmitted wave is left-handed circular polarization.
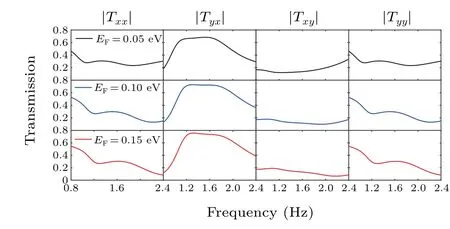
Figure 5 shows the four transmission matrices at different Fermi levels when the linearly polarized waves propagating in the backward direction. When the Fermi level of DSMs is modified from 0.05 eV to 0.15 eV,the amplitude of transmission coefficients are basically unchanged and the resonant frequency are blue shifted. Therefore, changing the Fermi level of the DSMs can achieve dynamic tunability of resonant frequency,thus increasing the applications of devices.
By analyzing the electric field of linearly polarized waves propagating in the backward direction, the AT effect can be more clearly understood. Figure 6(a)shows that the direction of the electric field changes from horizontal to vertical as thex-polarized waves are incident, which indicates that thex–ypolarization transition is achieved at 1.30 THz.In contrast,the direction of the electric field of the output wave is still vertical when they-polarized waves propagate,as shown in Fig.6(b).


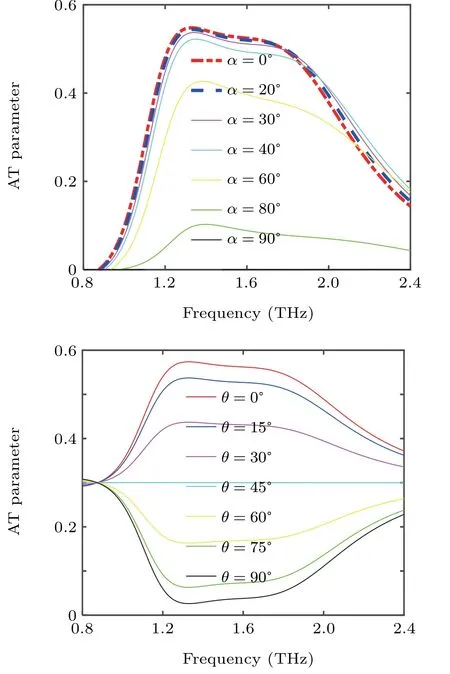
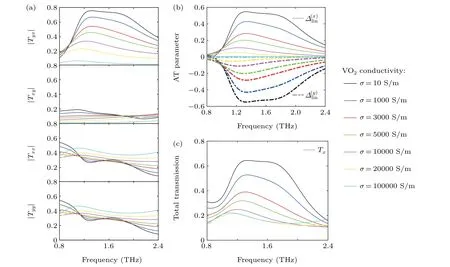
The intensity of the four transmission matrices can be tailored by modifying the conductivity of VO2as shown in Fig. 8(a). When thex- andy-linearly polarized waves propagate in the backward direction, the cross-polarized transmission coefficients|Tyx| and|Txy| decrease continuously as the metallic properties of VO2increase. However, the copolarized transmission coefficients|Txx|and|Tyy|increase continuously. Therefore,the curves in Fig.8(a)will form the phenomenon in Figs.8(b)and 8(c). It can be seen from Fig.8(b)that AT parameterΔxlincontinuously decreases by increasing the conductivity of the VO2. The phenomenon of AT basically disappears when the conductivity is 105S/m. With the conductivity of VO2increase,the rising conductivity leads to a continuous decrease in transmission, as shown in Fig. 8(c).Thus,AT occurs when VO2is in the insulator state and it can be turned off by increasing its conductivity.
4. Conclusion
In this work, we have realized a dynamically controlled broadband AT device for linearly polarized terahertz waves based on DSMs and VO2materials. When the VO2in its insulating state (10 S/m), the bandwidth of AT parameter greater than 0.5 is 0.59 THz in the frequency range of 1.21 THz–1.80 THz. When the VO2in its metallic state (105S/m),the AT parameter is almost 0 in the entire frequency range of 0.8 THz–2.4 THz. Therefore,the amplitude of AT can be dynamically tailored by modifying the conductivity of VO2. The resonant frequency of AT exhibits blue shift with the Fermi level of DSMs increases from 0.05 eV to 0.15 eV while maintaining the amplitude of AT.The method of adjusting resonant frequency and amplitude independently increases the practicability of devices,which is conducive to the development of AT devices.
Acknowledgments
Project supported by the National Natural Science Foundation of China (Grant Nos. 61875106, 62105187,and 61775123), the Natural Science Foundation of Shandong Province, China (Grant No. ZR2021QF010), the Shandong Social Science Planning Project, China (Grant No. 21CZXJ08), and the National Key Research and Development Program of China(Grant No.2017YFA0701000).
- Chinese Physics B的其它文章
- Switchable terahertz polarization converter based on VO2 metamaterial
- Data-driven parity-time-symmetric vector rogue wave solutions of multi-component nonlinear Schr¨odinger equation
- Neutron activation cross section data library
- Multi-phase field simulation of competitive grain growth for directional solidification
- A novel similarity measure for mining missing links in long-path networks
- Effects of electrical stress on the characteristics and defect behaviors in GaN-based near-ultraviolet light emitting diodes