張婷婷,魏廣生

(陜西師范大學(xué)數(shù)學(xué)與信息科學(xué)學(xué)院,陜西 西安 710062)

Stu rm-Liouv ille問題的特征值對勢函數(shù)的依賴性

張婷婷,魏廣生

(陜西師范大學(xué)數(shù)學(xué)與信息科學(xué)學(xué)院,陜西 西安 710062)

研究了定義在[0,1]上的Sturm-Liouville問題的特征值對勢函數(shù)的連續(xù)依賴性.應(yīng)用比較定理和定義區(qū)間單調(diào)性證明了:當(dāng)部分區(qū)間[x0,1]上的勢函數(shù)趨于無窮大時,[0,1]區(qū)間上的特征值漸進(jìn)趨近于[0,x0]區(qū)間上的某個特征值.推廣了一些作者對Sturm-Liouville問題研究的相應(yīng)結(jié)果,并為其相應(yīng)問題的研究提供了一個新的視角.

勢函數(shù);特征值;比較定理

1 引言

本文的動機(jī)由文獻(xiàn)[1-3]的工作所驅(qū)動.他們考慮了Sturm-Liouville(SL)問題的特征值依賴邊界,邊值條件以及勢函數(shù)的變化問題.所以SL問題是由微分方程:

另外,他們還研究了當(dāng)勢函數(shù)q,邊界條件和一個端點(diǎn)都固定時,SL問題的特征值和特征函數(shù)對另一個端點(diǎn)的依賴性.即當(dāng)區(qū)間長度向零收縮時,最小的Dirichlet特征值趨于無窮大,但這一結(jié)論對于最小的Neum ann特征值并不成立,因為他是一個有限極限.其后對SL問題的研究涌現(xiàn)出大量推廣性結(jié)論[4-7].

本文考慮上述問題(1)-(2)的特征值關(guān)于部分區(qū)間[x01]上的勢函數(shù)變化問題,證明了與其平行性的結(jié)論:當(dāng)[x01]區(qū)間上的勢函數(shù)趨于正無窮或負(fù)無窮大時,問題(1)-(2)的[0,1]區(qū)間上的特征值漸進(jìn)趨于[0,x0]上的某個特征值;并進(jìn)一步證明了上述問題(1)-(2)的[0,1]區(qū)間上的第m個特征值漸進(jìn)趨近于[0,x0]上的第m個特征值.

2 定理及證明

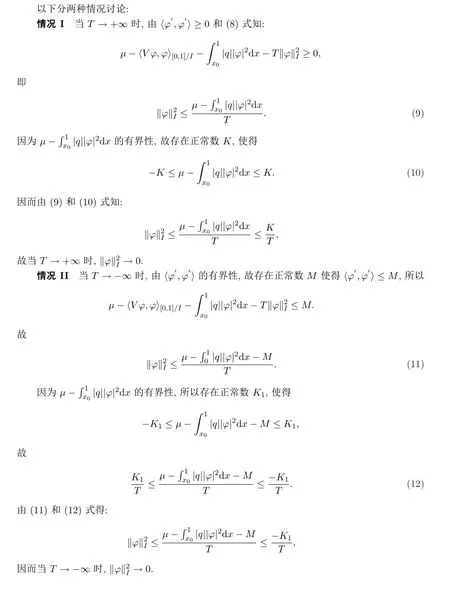
[1] Kong Q, Zettl A. Dependence of eigenvalues of Sturm-Liouville problems on the boundary[J]. Differential Equations, 1996,126:389-407.

[2] Kong Q, Zettl A. Eigenvalues of regular Sturm-Liouville problems[J]. Differential Equations, 1996,131:1-19.

[3] Kong Q, Zettl A. Linear ordinary differential equations in Inequalities and Applications"[J]. WSSIAA, 1994,3:381-384.

[4] Kong Q, Wu H, Zettl A. Dependence of the nth Sturm-Liouville Eigenvalue on the problem[J]. Differential Equations, 1999,156:328-354.

[5] Kong Q, Wu H, Zettl A. Dependence of eigenvalues on the problem[J]. Nachr. Math., 1997,188:173-201.

[6] Zettl A. Sturm-Liouville Problems in Spectral Theory and Computational Methods of Stur-Liouville Prob-lems"[M]. New York: Dekker, 1997.

[7]李麗,黃振友.一類Schr¨odinger算子的譜范圍[J].純粹數(shù)學(xué)與應(yīng)用數(shù)學(xué),2011,27(3):387-391.

[8] Evitan B M. On the determination of a Sturm-Liouvillle equation by two spectra[J]. Mat. Tidskr., 1949,B:25-30.

[9] Paul Phillips D. A partial inverse Sturm-Liouville problem: Matching a step function potential to a finite eigenvalue list[J]. Math. Anal. Appl., 2005,312:248-260.

[10] Chavel I. Eigenvalues in Riemannian Geometry[M]. Orlando: Academic Press, 1984.

Dependence of eigenvalues of Sturm-Liouville problems on the potential function

Zhang Tingting,WeiGuangsheng

(College of Mathematics and Information Science,Shaanxi Normal University,X i′an 710062,China)

This paper studiesmain ly the eigenvalues of Sturm-Liouville(SL)p roblem s def nded in[0,1]dependent continously on the potential function.By the com parison theorem and domainmonotonicity,it proves that when the potential function in the interval[x0,1]tends to in fnity,the eigenvalues in the interval[0,1]asym ptotically app roach the eigenvalues in the interval[0,x0].It promotes the corresponding results of Sturm-Liouville problems which some authors studies, and provides a new angle of view.

potential function,eigenvalue,comparison theorem

O175.3

A

1008-5513(2012)02-0232-06

2011-11-16.

國家自然科學(xué)基金(10771165).

張婷婷(1985-),碩士生,研究方向：微分算子的譜與逆譜理論.

2010 MSC:34A 55,34L24